


Next: The SU(2) Group
Up: The Standard Model of
Previous: Gauge Fields
Hadrons can be grouped in multiplets, for example
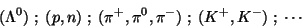 |
(176) |
defined by certain quantum numbers common to its members. All
the members of a given multiplete have the same 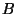 (baryon
number),
(baryon
number), 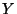 (hypercharge) (
(hypercharge) (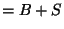 (strangeness)),
(strangeness)), 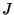 (spin) and differ by
the electric charge. Besides, it can be noticed that the masses of the
members of a given multiplet are almost equal, although not identical.
(spin) and differ by
the electric charge. Besides, it can be noticed that the masses of the
members of a given multiplet are almost equal, although not identical.
The strong interactions of the members of a given multiplet are
practically equal, for example the scattering amplitudes
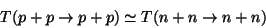 |
(177) |
this indicates that the nuclear forces are independent of the
electric charge of the interacting particles. In other words, in the
absence (hypothetic) of electromagnetic interactions there would be no
distinction between the proton state and the neutron state.
Summarizing, we find the existence of a symmetry indicating the
possibility of giving a classification group to assign the particles
the appropriated quantum numbers. Which group 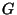 would it be? As the spin
is common to all the members of a multiplet, one has in principle
would it be? As the spin
is common to all the members of a multiplet, one has in principle
![\begin{displaymath}[G,Poincar\acute{e}]= 0
\end{displaymath}](img409.gif) |
(178) |
implying then that the masses are equal in the multiplet. Therefore, this
symmetry will be an approximated symmetry as this property is not fulfilled
exactly.
Subsections



Next: The SU(2) Group
Up: The Standard Model of
Previous: Gauge Fields
root
2001-01-22
![]() would it be? As the spin
is common to all the members of a multiplet, one has in principle
would it be? As the spin
is common to all the members of a multiplet, one has in principle